 If students are to understand mathematics, they must DO mathematics – the kind of doing that is much more than just working through algorithms comprised of meaningless steps for them.
Students must get their hands dirty, so to speak, and muck around with numbers, with number concepts, with problematic situations. They must try out strategies and ideas, talk about them, test them, prove them, and represent them. They must look for alternate strategies and process those in the same ways as the first strategies. They need to consider the ideas and strategies of others and compare those to their own. They need to look for generalizations among the ideas. They need to consider what new questions arise from their work.
Math can be “messy” as students work to make sense of problems. In fact, it can be argued that if it is not “messy”, then there really was no true problem at all. If one immediately knows how to solve something, there is no problem, just some figuring to be done. A true problem requires one search for a solution.
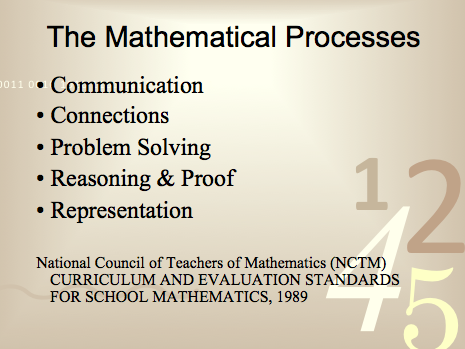
This all brings me to the mathematical processes that the NCTM has been promoting for many years: communication, connections, problem solving, reasoning and proof, and representation. When students are engaged regularly in these processes, they cannot help but build true understanding of mathematical concepts. Without the processes, there may be an ability to do calculations, but students will likely be devoid of any depth of understanding.
“Understanding ‘lives’ in the processes.” Norris C., S. Chorney, D. Wright, & T. Thielmann. “Communication on Communication”. Vector, Volume 53, Issue 1 (Spring 2012).
I hope you will put these processes into practice with your students.
Mathematically yours,
Carollee
If students are to understand mathematics, they must DO mathematics – the kind of doing that is much more than just working through algorithms comprised of meaningless steps for them.
Students must get their hands dirty, so to speak, and muck around with numbers, with number concepts, with problematic situations. They must try out strategies and ideas, talk about them, test them, prove them, and represent them. They must look for alternate strategies and process those in the same ways as the first strategies. They need to consider the ideas and strategies of others and compare those to their own. They need to look for generalizations among the ideas. They need to consider what new questions arise from their work.
Math can be “messy” as students work to make sense of problems. In fact, it can be argued that if it is not “messy”, then there really was no true problem at all. If one immediately knows how to solve something, there is no problem, just some figuring to be done. A true problem requires one search for a solution.
This all brings me to the mathematical processes that the NCTM has been promoting for many years: communication, connections, problem solving, reasoning and proof, and representation. When students are engaged regularly in these processes, they cannot help but build true understanding of mathematical concepts. Without the processes, there may be an ability to do calculations, but students will likely be devoid of any depth of understanding.
“Understanding ‘lives’ in the processes.” Norris C., S. Chorney, D. Wright, & T. Thielmann. “Communication on Communication”. Vector, Volume 53, Issue 1 (Spring 2012).
I hope you will put these processes into practice with your students.
Mathematically yours,
Carollee  If students are to understand mathematics, they must DO mathematics – the kind of doing that is much more than just working through algorithms comprised of meaningless steps for them.
Students must get their hands dirty, so to speak, and muck around with numbers, with number concepts, with problematic situations. They must try out strategies and ideas, talk about them, test them, prove them, and represent them. They must look for alternate strategies and process those in the same ways as the first strategies. They need to consider the ideas and strategies of others and compare those to their own. They need to look for generalizations among the ideas. They need to consider what new questions arise from their work.
Math can be “messy” as students work to make sense of problems. In fact, it can be argued that if it is not “messy”, then there really was no true problem at all. If one immediately knows how to solve something, there is no problem, just some figuring to be done. A true problem requires one search for a solution.
This all brings me to the mathematical processes that the NCTM has been promoting for many years: communication, connections, problem solving, reasoning and proof, and representation. When students are engaged regularly in these processes, they cannot help but build true understanding of mathematical concepts. Without the processes, there may be an ability to do calculations, but students will likely be devoid of any depth of understanding.
“Understanding ‘lives’ in the processes.” Norris C., S. Chorney, D. Wright, & T. Thielmann. “Communication on Communication”. Vector, Volume 53, Issue 1 (Spring 2012).
I hope you will put these processes into practice with your students.
Mathematically yours,
Carollee
If students are to understand mathematics, they must DO mathematics – the kind of doing that is much more than just working through algorithms comprised of meaningless steps for them.
Students must get their hands dirty, so to speak, and muck around with numbers, with number concepts, with problematic situations. They must try out strategies and ideas, talk about them, test them, prove them, and represent them. They must look for alternate strategies and process those in the same ways as the first strategies. They need to consider the ideas and strategies of others and compare those to their own. They need to look for generalizations among the ideas. They need to consider what new questions arise from their work.
Math can be “messy” as students work to make sense of problems. In fact, it can be argued that if it is not “messy”, then there really was no true problem at all. If one immediately knows how to solve something, there is no problem, just some figuring to be done. A true problem requires one search for a solution.
This all brings me to the mathematical processes that the NCTM has been promoting for many years: communication, connections, problem solving, reasoning and proof, and representation. When students are engaged regularly in these processes, they cannot help but build true understanding of mathematical concepts. Without the processes, there may be an ability to do calculations, but students will likely be devoid of any depth of understanding.
“Understanding ‘lives’ in the processes.” Norris C., S. Chorney, D. Wright, & T. Thielmann. “Communication on Communication”. Vector, Volume 53, Issue 1 (Spring 2012).
I hope you will put these processes into practice with your students.
Mathematically yours,
Carollee 